Sea State Forecasting

An Introduction to
SEA STATE FORECASTING
GRAHAM P. BRITTON
Captain, Royal Navy (Retired)
edited by: Kenneth E. Lilly, Jr.
Commander, NOAA
National Oceanic and Atmospheric Administration
National Weather Service
This report was prepared as an account of work sponsored by the U.S. Department of Commerce, Contract No. DOC 7-352] 8. Neither the United States, nor the National Oceanic and Atmospheric Administration, nor any person acting on behalf of the National Weather Service of NOAA: (A) Makes any warranty of representation: expressed or implied, in respect to the accuracy, completeness, or usefulness of the information contained in this report, or that the use of any information, apparatus, method, or process disclosed in this report may not infringe privately owned rights; or (B) Assumes any liabilities in respect to the use of any information, apparatus, method, or process
CHAPTER 10
WAVE STEEPNESS - FORECASTING SLAMMING AND HARBOR BAR CONDITIONS
10.1 Momentum of the sea surface layer. Many factors are involved in the development of waves: the speed and acceleration of the wind, the state of the atmosphere, and the sea surface temperature. But there is another factor which should be taken into account -the existing momentum of the upper layers of the ocean since this has an important bearing on the drag coefficient and the degree of bite that the wind can exercise.
During wave generation, air moving over the surface of the sea at higher velocity than the waves will flow more easily if the surface itself has some velocity in the same direction. But if it has a contrary velocity, the lighter air mass is partially and periodically arrested by impacting the much denser water surface. Momentum is exchanged by a degree of impact. The turbulence in the bottom layer increases, allowing the air mass to get even more bite.
The above explanation may not be entirely adequate, but it serves to explain certain facts observed by seamen such as that gustiness changes with the changing of a tide due to the change of attitude of the wind to the waves. The potential danger of a tidal change is one which is well known to the yachtsman and the small boat fisherman. When a strong wind is blowing with a tide, maneuvering and operating fishing gear, although difficult, may not be impossible. A wind of the same force blowing against the tide will cause the waves to stand up or mushroom and may swamp a small boat. Good seamen take precautions in estuaries and coastal waters where tidal currents run strongly. But this is not solely a coastal problem, and the principles apply equally to the case of currents in the open oceans.
It is important for a forecaster, particularly in closed and semi-closed seas, to remember that wave height increases at a faster rate than wave length irrespective of current flow. The work of Darbyshire has shown that almost all growth of wave height occurs when the fetch is less than 100 miles. This is particularly true in cases of air mass/sea surface temperature classification I (see Chapter 7, Figure 26[1]).
Neither the empirical nor the classical formulas cater to the growth rate adequately and, in particular, do not take into account existing current or tidal flow. Yet the matter can be one of critical importance to small ships, such as trawlers.
10.2 Contrary currents to winds in the normal Gulf of Alaska. In Chapter 61, the subject of "holes" in the ocean was discussed. In particular, reference was made to the Agulhas Bank off the southern shores of Africa where the prevailing wind blows contrary to the Agulhas current over a continental shelf with a pronounced sill, all things combining to make the waters particularly dangerous to shipping. It is not without reason that sailors called the Cape of Good Hope by another name -the Cape of Storms
Similar circumstances arise in the Gulf of Alaska and along the Aleutian Chain where the damage to ships annually is very high. Depressions moving eastwards over the North Pacific often slow down and deepen in the Gulf, their progress arrested by blocking anticyclones positioned over the land masses to the north and east. This is particularly true in autumn and winter when the continental anticyclones intensify over the frozen land masses. The shape of the Gulf aids the process. The circulations around the depressions that remain and deepen generate and reinforce the counterclockwise flow of surface water northwards along the coast of British Columbia and then westwards along the southern shores of Alaska. This current is an established climatic feature of the general circulation and is reflected in the pattern of the sea surface isotherms. In this area of ocean, current data is based on a relatively few number of observations, and the current atlases need to be improved. Although the average westerly set is only of the order of 1.0 knot for most of the year, the surface flow of water may be accentuated if deep depressions remain almost stationary for several days. A synoptic current is created to reinforce the climatic one.
When such current flow is well established any outbreak of cold dry arctic air from off Alaska in winter on the Gulf area will cause the rapid growth of steep waves having short wave lengths. Close to the coast, ice accretion on superstructures and rigging resulting from freezing spray becomes an added hazard, and a ship may suddenly be in considerable danger.
The factors contributing to steep seas have been defined above, but how is the meteorologist who has seldom had training or experience in currents and tides to cope? He would not normally consider taking currents into account in a forecast; no empirical formulas or diagnoses exist to help him. He should derive as much information as is available from current and tidal atlases. If time is available, wind vector diagrams should be kept for fixed positions in the ocean, such as the buoy positions, and from these it can be readily seen by eye how the wind has been blowing relative to the direction of current set over the last week or ten days.
The problem is an urgent one which requires research. When automatic buoy arrays have finally been established in the coastal waters of the Gulf of Alaska, some current data may result which will help resolve the problems and determine the rate of growth of seas in unusual circumstances. Until such time as buoys can supply current data it can only be suggested that forecasters bear this important operational hazard in mind, using with caution the modifications to recognized methods, which will now be suggested.
10.3 Changes in the steepness factor caused by currents or tides and ship's speed. The steepness of the waves is often of far greater interest to the seaman than the mere heights of the waves, and it is important for the seaman to appreciate how steepness will vary with the speed of a current and the speed of the ship relative to the waves. The latter factors are only known aboard the ship and provide one good reason for the mariner himself to understand the problem and undertake some interpretation on board.
A ship steering into incoming seas will meet more wave lengths in any given time interval than she would if either stationary or moving with the waves. This brings about a relative or apparent increase in wave steepness. The effect is very noticeable in a power boat, accelerating away from a beach. The boat soon begins to bounce on the waves.
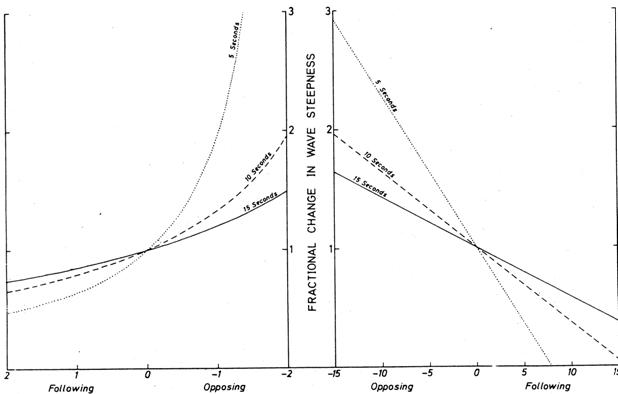
Similarly, when a current is moving against the sea, an increase in wave steepness results. The relative increases in wave steepness are given in Figure 34 (currents) and Figure 35 (ship's speed). The two effects are cumulative. The graphs represent the fractional increases in steepness due to the speed of the current or the speed of the ship.
Example I - From Figure 34 we see that when a current of 1.5 meters/second (three knots) is flowing opposite to the sea and swell, the steepness of the waves will be increased by approximately 40% in a swell of period 15 seconds and by approximately 70% in a swell of period 10 seconds. In a sea of five seconds, it will be increased at least three or four times, which would almost certainly cause such short period waves to become top heavy and break. Current speeds of 1.5 meters/second (three knots) are not abnormal in tidal waters. Hence, the figures quoted are very realistic. The broken water in any tide rip illustrates the point.
Example II - Similarly, from Figure 35, a ship proceeding at 10 meters/second (20 knots) into a swell of period 15 seconds will experience an apparent increase of steepness of 50%; 70% in seas or swells of 10 second period; and 150% in seas of six second period. Such apparent increases in steepness will affect ships in different ways according to their dimensions. Small ships and power boats would certainly experience slamming as the result of the increase in steepness of the seas, whereas cargo vessels and passenger ships of considerable length might be forced to slow down in longer period swells.
When both factors are present, the product of the two effects results. Thus a ship heading at 15 knots aided by a tide or current of three knots into a swell of period 10 seconds could experience an apparent increase of steepness of 1.7 x 1.7 (i.e., almost three times). Such circumstances might arise when a ship emerges from a river mouth or port entrance into an oncoming sea or swell. This could well force the ship to reduce speed to avoid slamming. Wave periods of 10 seconds are not uncommon in open waters; thus, it is an important matter for ships to be aware of the problem and consult current data.
Current and tidal atlases are barely adequate for the purpose, and much available current data remains to be incorporated in them. To some degree, current flow is indicated by features of bottom topography, and there is often considerable difference in the sea conditions experienced in the shallow waters close to the coastline and the deeper waters further to seaward. There is little likelihood that these factors will be incorporated in sea and swell models and so it is important for the seamen to take them into his own considerations of the problem.
10.4 Estuary and harbor bar forecasting. So many boating and fishing tragedies occur each year at holiday times at or near the mouths of river estuaries that the subject merits special comment. To the weather forecaster, who has the task of issuing weather forecasts for such areas, it imposes a special challenge and demands his highest skills and experience as a professional person. Unfortunately, most forecasters seldom receive adequate training to be able to tackle the complexities of such problems. On any shore the marine forecaster, given the aids of synoptic charts, satellite data, and so on, will normally have the capability to make predictions of sea state conditions a few miles offshore before the waves begin to feel bottom. With a little local experience and after some study of the bottom topography, he can usually derive from these data a surf forecast for the beaches sufficient to give holiday makers some indication of when dangerous breaking waves are likely to be experienced. Little more should be expected of him; although this should be a minimal requirement of all weather forecasters engaged in marine forecasting. The problem will receive various degrees of attention by weather services according to the exposure of the coast. In many cases, but by no means all, the greatest impact occurs in temperate latitudes on the westward side of the major continents where there is a long fetch to windward in the westerlies
But at, or near, the mouths of river estuaries the problems created by surf are considerably increased as a result of the current flow from the river mouth, augmented at certain times of day by the ebb tide. When these act in maximum conjunction at the height of the ebb (or shortly afterwards) and in direct opposition to the onshore movement of seas and swells, in which the steepness of the waves increases automatically with shoaling, the sea state situation may deteriorate rapidly to place small craft in considerable jeopardy. In many instances this is such a localized and specialized problem requiring and experience that the regional weather forecast is only of secondary consideration to the fishermen. But it need not be so, for with good training and some special attention to the problem, marine forecasting services have something to offer to the fishing community, not only to enable them to program sailing times more efficiently, but in the worst circumstances, make a contribution to the safety of boats and men.
The specialized knowledge of the fishermen, the local pilots, and the Coast Guard is not always reflected in the weather forecasts for these areas, and hence, many warnings of dangerous sea state circumstances do not reach the general public. .Many weather forecasters might even oppose the suggestion that they be given any responsibility in this matter since the problem is so specialized in its nature and depends considerably on knowledge of river currents, tides, and ship characteristics.[2] It must be acknowledged that they have some point, for without trainings, the problem can barely be attempted.
10.5 The basic requirements for a sea state forecast at a river mouth. The first requirement is the determination of the maximum speed of the stream due to the combination of current and tide. The speed of many rivers is now control1ed by water authorities and such information is often readily available. However, there may be occasions following heavy rainfall or flooding when the river speeds are above normal, and the forecaster will have to use local observations and perhaps exercise personal judgement
Tidal information for all major ports and secondary ports is found in the Tide Tables and is generally sufficient to obtain the times of high and Jaw water and the range of the tide. The proximity to springs or neaps, when tidal effects are near their maximum or minimum, respectively, is also readily available. Specific information on tidal currents is contained in the Tidal Current Tables, which are issued for each year. The combination of ebb tide and river current speed may well exceed six knots.
It is a more difficult matter for the forecaster to be up-to-date with the actual flow patterns of the stream in the vicinity of the harbor mouth and over the harbor bar itself, for these are susceptible to continual change and, occasionally, radical alteration following a major storm. Silting is often a considerable problem in these localities requiring channels to be either periodically or regularly dredged. Any major constrictions of the channel due to silting or the deposition of sand or pebbles will cause the maximum current to be augmented in some areas and reduced in others. Generally, the water flow off the entrance results in complex eddy motions for a considerable distance seaward. Sometimes this is plainly seen in satellite pictures, particularly the enhanced infra-red images when the water temperature of the sea and river water have some degree of separation.
The forecaster needs some knowledge of the river and eddy flows, which in most circumstances can be obtained from officials in charge of coastal engineering. If the river traffic is considerable and has major economic significance, it is likely that scale models of the harbor have been constructed to investigate the problems of silting. Familiarization with the results obtained from such models, and some experiments with sea, swell, and wind inputs could prove a tremendous help to a forecaster.
A basic requirement is the latest knowledge of the wave spectra or an estimation of the frequency and height distributions in the sea a few miles offshore in deep water before the waves have begun to feel bottom. Once again this is information which will not normally be readily available, and the forecaster will need to deduce it from the latest synoptic charts. In any event, there will be a forecasting task to predict what the conditions will be at the time of the subsequent ebb tide or tides.
The main contribution from the weather forecaster could well be the accurate prediction of the local weather change and particularly the development of a system off the coast which will enhance the onshore flow.
In particular, the forecaster should make allowance for the sea breeze effects in the afternoons, which on certain coastlines of the world can produce appreciable wind speeds for a few hours. If this flow enhances the synoptic flow there will be considerable increase in waves of short period, which are particularly prone to the effects of adverse currents. The effects on ships of large tonnage will not be great, but it will greatly affect fishing vessels and pleasure craft.
10.6 Theoretical considerations. Theoretically, the period T is a conservative quantity (see Appendix A1), and we have the equations:
- where C = the speed of the wave in deep water,
- L = the wave length of the wave in deep water,
- c = the speed of the wave in shallow water,
- l = the wave length of the wave in shallow water.
As the waves approach a shore, some refraction takes place, and the speed of the waves decreases as the waves in the rear of a train start to catch up with those in front. This results in more wave energy becoming concentrated into a smaller area. Relatively, the potential energy increases; hence, the wave height tends to increase.
Since we are dealing with the shallow water case, tanh(2πd/L) approximates to 2πd/L. Hence:
Equations (10.1), (10.2), and (10.3) will hold generally for coastal waters, but will need some modification at river and estuary entrances where there is an current or ebb tide of appreciable magnitude.
The period T will not remain conservative j and the general effect will be to cause some increase in the reciprocal value. Waves of short period will be affected more so than the waves of longer period, and the wave lengths will decrease correspondingly.
Hence, if we consider equation (10.3), it is seen that wave steepness is increased for each of the three considerations:
- (a)
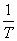 tends to increase, the stronger the opposing current or tide.
tends to increase, the stronger the opposing current or tide.
- (b)H tends to increase as more potential energy is crowded into a smaller area.
- (c)
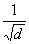 tends to increase as the depth of water decreases.
tends to increase as the depth of water decreases.
The ratio of height to wave length will steadily increase until the ratio approaches 1/10. At this ratio, some of the higher waves will be breaking, and theoretically, all should be breaking when the ratio H/L = 1/7.
Every entrance poses a different problem on account of the topography, especially the depth of water required over the harbor bar to allow for ready access to shipping. In the case of the Columbia River entrance, the channel is dredged to between 40 and 50 feet. Some rules for forecasting breaking sea conditions for the Columbia River bar have been developed. Those rules appear to work well and could well serve as a model for similar river mouths.
Low level photography of swells approaching the shore or a river entrance can be most instructive and are well illustrated in Plates 71 and 81 of Alsea Bay and Coquille River in Oregon. The short steep breaking waves in the foreground of Plate 7 clearly illustrate the results of the water outflow acting against the incoming swell. There are no jetties, and the sand shifts frequently in an unstable entrance. A small boat with an inexperienced crew could easily be in difficulties in trying to enter or leave this river mouth.
Plate 8 shows clearly that certain areas are more dangerous than others. Note in particular how the waves are breaking on the shores outside the jetties and the confused state of the sea close to the jetty on the right of the picture. The areas in the neighborhood of both jetties are to be avoided as far as possible.
|
Figure 34 - Effect of current on wave steepness. (From "High Waves in the Agulhas Current" by E.H. Schumann. Mariners Weather Log, January 1976) |
Figure 35 - Effect of ship's speed on wave steepness. (From "High Waves in the Agulhas Current" by E.H. Schumann. Mariners Weather Log, January 1976.) |
[1] Not provided in this web page.
[2] Ed. note: In 1980, routine forecasts for the bar at Grays Harbor, Washington and the mouth of the Columbia River in Oregon were being issued by the NWS.


